Physics and Astronomy Labs/Heisenberg's uncertainty and Beethoven's fugue
Review of the uncertainty principle
edit- This gallery contains images and discussions of Heisenberg's uncertainty principle and other topics pertinent to this lab. Be sure to click the links to Wikimedia commons because the images contain detailed discussion.
Introducing the specimen to be studied
editThe third movement of Beethoven's fifth symphony[1] contains within it a fugue that begins with the cellos playing:[2]
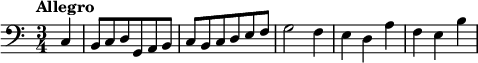
For those of you unfamiliar with musical notation, this score informs us that the music is to be played at rate of 96 bars every minute. The fast notes are called eighth notes, and there are six such notes in every bar.
I think the first note is one octave below middle C
Two new files on commons
edit
This audo file can be studied if you download http://audacity.sourceforge.net/download/
Why do cellos and other bass instruments sound "muddy" when they play fast?
The following explanation may or may not explain why. It appeared in a homework assignment I saw back in the 1970s. On the other hand, people have told me that it is not a very rigorous argument. It's not on Wikipedia, and there are few websites that discuss this topic.
- See also the new image on Heisenberg's microscope
Calculation
editWe begin with a calculation based on a website at http://newt.phys.unsw.edu.au/jw/uncertainty.html
A tone burst is a pure pitch at some frequency that lasts for a short duration, as shown in the figure. A simple way to look at the uncertainty principle is to recognize that tone bursts usually start gradually, not as the "perfect" sine wave, f(t)=Asinωt. Suppose that even the best tone burst creates an uncertainty of one cycle in the number of cycles that occur in the number of cycles that occur in a time of duration, τ. Letting N be the number of cycles, we have for the frequency,
Hence,
A bit of confusion
editA different calculation appears on a website by http://www.csulb.edu/~kmerry/FourierAnalysis/Fourier_14.pdf
The choice of 4π is made to match a theoretical minimum uncertainty achieved using advanced mathematics. It leads to a minimum uncertainty:
The 12-tone scale: Divide a factor of 2 into 12 equal parts
editThe piano divides the octave into 12 even parts, in what is known as w:equal temperament. Since , we see that adjacent notes are about 6% apart. For high pitched notes, 6% of f is a large number, much larger than the "muddiness" implied by the uncertainty principle. But for low pitch notes, the difference between adjacent notes is much smaller. Perhaps it is even smaller than the uncertainty in pitch. Define the difference between adjacent notes on the 12-tone scale as,
Equating to would give us the transition frequency, where the uncertainty in pitch exceeds the difference in pitch between neighboring notes.
Violins in an orchestra tune their second highest open string to a standard pitch of 440A. The cello's open A string is an octave lower, and this is the cellos highest open string. Cellos can easily go an octave lower than that. Therefore a reasonable "low" note for cellos is at 100 Hertz
Today's lab
edit- Listen to the fugue and estimate the time duration of the fast notes, this duration is called 'tau', .
- The class got 10 bars in seven seconds. The period is:7/60 = 0.11 sec = τ
- Middle C is 261.6 Hz and the cellos started one octave lower, so:
- The first note was at f = 131 Hz.
- Using the first definition for uncertainty Δf = 1/τ = 9.1 Hz.
- Δf/f = 9.11/131 = .069 ≈ 7%
- Adjacent notes on a piano are 6% apart because a piano divides the octave (factor of two) into 12 even parts: 21/12 = 1.059 ≈ 1 + .06 = 1+6%.
- Therefore the notes are right at the muddy threshold for if the uncertainty in the number of cycles is 1. But, the strict mathematical limit reduces this uncertainty by a factor of 4π≈13, so by this criteria the cellos should not be muddy.
- Come up with a good estimate of the typical low note frequency (f=100Hz is a good starting point).
- Compare the uncertainty in the notes' pitch to the difference between adjacent pitches.
- At 100 Hz, the difference in pitch between adjacent notes is ________
- In Beethoven's fugue, the "muddiness" in pitch is ________
- Which is bigger, the "muddiness" or the difference in pitch between adjacent notes in the bass instruments?
- Now repeat the calculation with some exact numbers.
There is not much on the internet
edit- http://newt.phys.unsw.edu.au/jw/uncertainty.html Quality page. The only on that we are certain we can use.
- http://www.csulb.edu/~kmerry/FourierAnalysis/Fourier_14.pdf Another page that confirms what I heard as a student at Berkeley
- https://www.youtube.com/watch?v=TG9jIdIPeNA Does not seem serious, but I did not go to the end
- http://www.thephysicsmill.com/2013/01/13/resolution-fourier-analysis-and-the-heisenberg-uncertainty-principle/ Looks correct but seems too cmplicated
- http://knutsacoustics.com/files/Onset-transient-times-Rev.2.pdf a serious but apparently unrefereed article
Footnotes and references
edit- ↑ A public domain recording is available at http://imslp.org/wiki/Symphony_No.5,_Op.67_%28Beethoven,_Ludwig_van%29
- ↑ Go the the bottom of page 5 of this open source score








