Full octahedral group




The full octahedral group Oh is the hyperoctahedral group of dimension 3. This article mainly looks at it as the symmetry group of the cube.
There are ![]() A000165(3) = 48 permutation of the cube. Half of them are its rotations, forming the subgroup O (the symmetric group S4), and the other half are their inversions.
A000165(3) = 48 permutation of the cube. Half of them are its rotations, forming the subgroup O (the symmetric group S4), and the other half are their inversions.
The inversion is the permutation that exchanges opposite vertices of the cube. It is not to be confused with the inversion of a permutation.
| Elements of O | Inversions of elements of O | ||||
|---|---|---|---|---|---|
| identity | neut | 0 | inversion | inv3 | 0' |
| 3 × rotation by 180° about a 4-fold axis | inv2 | 7, 16, 23 | 3 × reflection in a plane perpendicular to a 4-fold axis | ref1 | 7', 16', 23' |
| 8 × rotation by 120° about a 3-fold axis | rot3 | 3, 4, 8, 11, 12, 15, 19, 20 | 8 × rotoreflection by 60° | rotref3 | 3', 4', 8', 11', 12', 15', 19', 20' |
| 6 × rotation by 180° about a 2-fold axis | rot2 | 1', 2', 5', 6', 14', 21' | 6 × reflection in a plane perpendicular to a 2-fold axis | ref2 | 1, 2, 5, 6, 14, 21 |
| 6 × rotation by 90° about a 4-fold axis | rot1 | 9', 10', 13', 17', 18', 22' | 6 × rotoreflection by 90° | rotref1 | 9, 10, 13, 17, 18, 22 |
| Examples | ||||
|---|---|---|---|---|
 |
 |
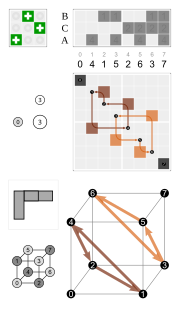 |
 |
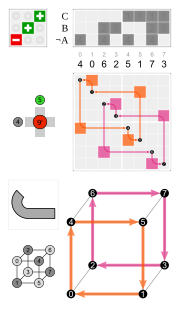 |
| neut | inv2 | rot3 | rot2 | rot1 |
| inv3 | ref1 | rotref3 | ref2 | rotref1 |

|

|

|

|

|
| See full list below: 8×6 matrix or Conjugacy classes | ||||
As the hyperoctahedral group of dimension 3 the full octahedral group is the wreath product ,
and a natural way to identify its elements is as pairs with and .
But as it is also the direct product , one can simply identify the elements of tetrahedral subgrup Td as and their inversions as .
So e.g. the identity is represented as and the inversion as .
is represented as and as .
A rotoreflection is a combination of rotation and reflection.
While a rotation leaves its axis and a reflection leaves its plane unchanged, a rotoreflection leaves only the center unchanged.
| Illustration of rotoreflections | ||||
|---|---|---|---|---|
|
| ||||
|
| ||||
Cayley table
editAs there are two ways to denote the elements of this group, there are two ways to write the Cayley table.
S4
editThe easier one is that in S4 based notation: It simply doubles the Cayley table of S4.
With and being their respective inversions, implies and .
Example:
pairs
editThe notation with pairs is probably more useful, but also more complicated:
The calculation of involves the cube vertex permutation shown in the images. E.g. .
simply follows from the Cayley table of S3:
Example:
| example | |||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
|
Overview
editTruncated cuboctahedron
editThe vertices of the truncated cuboctahedron correspond to the elements of this group. Each of its faces of its dual, the disdyakis dodecahedron, is a fundamental domain.

|

|

|

|
8×6 matrix
edit
|
Hexagon corresponding to top matrix row
edit| 3D diagrams | |||||
|---|---|---|---|---|---|
|
The files below illustrate the subgroup C3v or [3] that corresponds to the top matrix row. It contains the six permutations of the cube that leave the main diagonal fixed.
|
| 2D equivalents | ||||
|---|---|---|---|---|
|
The rest of this article uses left action, i.e. means first , then ,
|
| details | |||||||
|---|---|---|---|---|---|---|---|
 |
| ||||||
| This is left action again, so the 3×3 permutation matrices shown here are the transposes of those in the small permutohedron in the box above - which only makes a difference for | |||||||
Cubes corresponding to matrix columns
editEach of the six cubes in the following collapsible boxes shows one of the basic permutations from the top row of the matrix in the bottom left position.
In the other seven positions are the products of applying the reflections along coordinate axes on these basic permutations.
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Conjugacy classes
editThe full octahedral group has ![]() A000712(3) = 10 conjugacy classes.
A000712(3) = 10 conjugacy classes.
Two permutations and are complementary to each other, if .
Complementary permutations sum up to a vector of 7s, and their inversion sets are complements,
so their inversion numbers sum up to 28. (Compare one of the number matrices above.)
The conjugacy classes below are always shown in complementary pairs (like inv2/ref1 or rot2/ref2).
The numbers over the triangles are the inversion numbers of the corresponding permutations. It can be seen that corresponding numbers add up to 28.
| neut (1) | inv3 (1) | inv2 (3) | ref1 (3) | rot3 (8) | rotref3 (8) | rot2 (6) | ref2 (6) | rot1 (6) | rotref1 (6) |
|---|---|---|---|---|---|---|---|---|---|

|

|
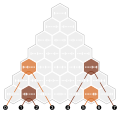
|

|

|
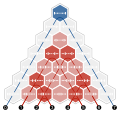
|
|

|

|

|
| neut (1 × 1) | inv3 (1 × 1) |
|---|---|
0
|
28
|
 |
 |
| inv2 (3 × 1) | ref1 (3 × 1) | ||||
|---|---|---|---|---|---|
12
|
20
|
24
|
16
|
8
|
4
|
 |
 |
 |
 |
 |
 |
| rot3 (4 × 2) | |||||||
|---|---|---|---|---|---|---|---|
6
|
14
|
18
|
18
| ||||
 |
 |
 |
 |
 |
 |
 |
 |
| rotref3 (4 × 2) | |||||||
|---|---|---|---|---|---|---|---|
22
|
14
|
10
|
10
| ||||
 |
 |
 |
 |
 |
 |
 |
 |
| rot2 (6 × 1) | |||||
|---|---|---|---|---|---|
26
|
18
|
20
|
12
|
24
|
8
|
 |
 |
 |
 |
 |
 |
| ref2 (6 × 1) | |||||
|---|---|---|---|---|---|
2
|
10
|
8
|
16
|
4
|
20
|
 |
 |
 |
 |
 |
 |
| rot1 (3 × 2) | rotref1 (3 × 2) | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
6
|
12
|
12
|
22
|
16
|
16
| ||||||
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
| Conjugacy classes of square permutations | |||||||
|---|---|---|---|---|---|---|---|
| neut | inv2 | ref1 | ref2 | rot1 | |||
0
|
6
|
2
|
4
|
1
|
5
|
3
| |
 |
 |
 |
 |
 |
 |
 |
 |
| Examples for tesseract and penteract |
|---|
|
The respective numbers of conjugacy classes for 4 and 5 dimensions are 20 and 36. examples4 = {
(0, 0): (0, 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12, 13, 14, 15),
(0, 1): (0, 1, 2, 3, 8, 9, 10, 11, 4, 5, 6, 7, 12, 13, 14, 15),
(0, 3): (0, 1, 4, 5, 8, 9, 12, 13, 2, 3, 6, 7, 10, 11, 14, 15),
(0, 7): (0, 2, 1, 3, 8, 10, 9, 11, 4, 6, 5, 7, 12, 14, 13, 15),
(0, 9): (0, 2, 4, 6, 8, 10, 12, 14, 1, 3, 5, 7, 9, 11, 13, 15),
(1, 0): (1, 0, 3, 2, 5, 4, 7, 6, 9, 8, 11, 10, 13, 12, 15, 14),
(1, 1): (1, 3, 0, 2, 5, 7, 4, 6, 9, 11, 8, 10, 13, 15, 12, 14),
(1, 2): (1, 0, 3, 2, 9, 8, 11, 10, 5, 4, 7, 6, 13, 12, 15, 14),
(1, 3): (1, 3, 5, 7, 0, 2, 4, 6, 9, 11, 13, 15, 8, 10, 12, 14),
(1, 7): (1, 3, 0, 2, 9, 11, 8, 10, 5, 7, 4, 6, 13, 15, 12, 14),
(1, 8): (1, 0, 5, 4, 9, 8, 13, 12, 3, 2, 7, 6, 11, 10, 15, 14),
(1, 9): (1, 3, 5, 7, 9, 11, 13, 15, 0, 2, 4, 6, 8, 10, 12, 14),
(3, 0): (3, 2, 1, 0, 7, 6, 5, 4, 11, 10, 9, 8, 15, 14, 13, 12),
(3, 2): (3, 2, 7, 6, 1, 0, 5, 4, 11, 10, 15, 14, 9, 8, 13, 12),
(3, 6): (3, 2, 1, 0, 11, 10, 9, 8, 7, 6, 5, 4, 15, 14, 13, 12),
(3, 8): (3, 2, 7, 6, 11, 10, 15, 14, 1, 0, 5, 4, 9, 8, 13, 12),
(3, 16): (3, 7, 11, 15, 2, 6, 10, 14, 1, 5, 9, 13, 0, 4, 8, 12),
(7, 0): (7, 6, 5, 4, 3, 2, 1, 0, 15, 14, 13, 12, 11, 10, 9, 8),
(7, 6): (7, 6, 5, 4, 15, 14, 13, 12, 3, 2, 1, 0, 11, 10, 9, 8),
(15, 0): (15, 14, 13, 12, 11, 10, 9, 8, 7, 6, 5, 4, 3, 2, 1, 0)
}
examples5 = {
(0, 0): (0, 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12, 13, 14, 15, 16, 17, 18, 19, 20, 21, 22, 23, 24, 25, 26, 27, 28, 29, 30, 31),
(0, 1): (0, 1, 2, 3, 4, 5, 6, 7, 16, 17, 18, 19, 20, 21, 22, 23, 8, 9, 10, 11, 12, 13, 14, 15, 24, 25, 26, 27, 28, 29, 30, 31),
(0, 3): (0, 1, 2, 3, 8, 9, 10, 11, 16, 17, 18, 19, 24, 25, 26, 27, 4, 5, 6, 7, 12, 13, 14, 15, 20, 21, 22, 23, 28, 29, 30, 31),
(0, 7): (0, 1, 4, 5, 2, 3, 6, 7, 16, 17, 20, 21, 18, 19, 22, 23, 8, 9, 12, 13, 10, 11, 14, 15, 24, 25, 28, 29, 26, 27, 30, 31),
(0, 9): (0, 1, 4, 5, 8, 9, 12, 13, 16, 17, 20, 21, 24, 25, 28, 29, 2, 3, 6, 7, 10, 11, 14, 15, 18, 19, 22, 23, 26, 27, 30, 31),
(0, 27): (0, 2, 1, 3, 8, 10, 9, 11, 16, 18, 17, 19, 24, 26, 25, 27, 4, 6, 5, 7, 12, 14, 13, 15, 20, 22, 21, 23, 28, 30, 29, 31),
(0, 33): (0, 2, 4, 6, 8, 10, 12, 14, 16, 18, 20, 22, 24, 26, 28, 30, 1, 3, 5, 7, 9, 11, 13, 15, 17, 19, 21, 23, 25, 27, 29, 31),
(1, 0): (1, 0, 3, 2, 5, 4, 7, 6, 9, 8, 11, 10, 13, 12, 15, 14, 17, 16, 19, 18, 21, 20, 23, 22, 25, 24, 27, 26, 29, 28, 31, 30),
(1, 1): (1, 3, 0, 2, 5, 7, 4, 6, 9, 11, 8, 10, 13, 15, 12, 14, 17, 19, 16, 18, 21, 23, 20, 22, 25, 27, 24, 26, 29, 31, 28, 30),
(1, 2): (1, 0, 3, 2, 5, 4, 7, 6, 17, 16, 19, 18, 21, 20, 23, 22, 9, 8, 11, 10, 13, 12, 15, 14, 25, 24, 27, 26, 29, 28, 31, 30),
(1, 3): (1, 3, 5, 7, 0, 2, 4, 6, 9, 11, 13, 15, 8, 10, 12, 14, 17, 19, 21, 23, 16, 18, 20, 22, 25, 27, 29, 31, 24, 26, 28, 30),
(1, 7): (1, 3, 0, 2, 5, 7, 4, 6, 17, 19, 16, 18, 21, 23, 20, 22, 9, 11, 8, 10, 13, 15, 12, 14, 25, 27, 24, 26, 29, 31, 28, 30),
(1, 8): (1, 0, 3, 2, 9, 8, 11, 10, 17, 16, 19, 18, 25, 24, 27, 26, 5, 4, 7, 6, 13, 12, 15, 14, 21, 20, 23, 22, 29, 28, 31, 30),
(1, 9): (1, 3, 5, 7, 9, 11, 13, 15, 0, 2, 4, 6, 8, 10, 12, 14, 17, 19, 21, 23, 25, 27, 29, 31, 16, 18, 20, 22, 24, 26, 28, 30),
(1, 26): (1, 0, 5, 4, 3, 2, 7, 6, 17, 16, 21, 20, 19, 18, 23, 22, 9, 8, 13, 12, 11, 10, 15, 14, 25, 24, 29, 28, 27, 26, 31, 30),
(1, 27): (1, 3, 5, 7, 0, 2, 4, 6, 17, 19, 21, 23, 16, 18, 20, 22, 9, 11, 13, 15, 8, 10, 12, 14, 25, 27, 29, 31, 24, 26, 28, 30),
(1, 31): (1, 3, 0, 2, 9, 11, 8, 10, 17, 19, 16, 18, 25, 27, 24, 26, 5, 7, 4, 6, 13, 15, 12, 14, 21, 23, 20, 22, 29, 31, 28, 30),
(1, 32): (1, 0, 5, 4, 9, 8, 13, 12, 17, 16, 21, 20, 25, 24, 29, 28, 3, 2, 7, 6, 11, 10, 15, 14, 19, 18, 23, 22, 27, 26, 31, 30),
(1, 33): (1, 3, 5, 7, 9, 11, 13, 15, 17, 19, 21, 23, 25, 27, 29, 31, 0, 2, 4, 6, 8, 10, 12, 14, 16, 18, 20, 22, 24, 26, 28, 30),
(3, 0): (3, 2, 1, 0, 7, 6, 5, 4, 11, 10, 9, 8, 15, 14, 13, 12, 19, 18, 17, 16, 23, 22, 21, 20, 27, 26, 25, 24, 31, 30, 29, 28),
(3, 2): (3, 2, 7, 6, 1, 0, 5, 4, 11, 10, 15, 14, 9, 8, 13, 12, 19, 18, 23, 22, 17, 16, 21, 20, 27, 26, 31, 30, 25, 24, 29, 28),
(3, 6): (3, 2, 1, 0, 7, 6, 5, 4, 19, 18, 17, 16, 23, 22, 21, 20, 11, 10, 9, 8, 15, 14, 13, 12, 27, 26, 25, 24, 31, 30, 29, 28),
(3, 8): (3, 2, 7, 6, 11, 10, 15, 14, 1, 0, 5, 4, 9, 8, 13, 12, 19, 18, 23, 22, 27, 26, 31, 30, 17, 16, 21, 20, 25, 24, 29, 28),
(3, 16): (3, 7, 11, 15, 2, 6, 10, 14, 1, 5, 9, 13, 0, 4, 8, 12, 19, 23, 27, 31, 18, 22, 26, 30, 17, 21, 25, 29, 16, 20, 24, 28),
(3, 26): (3, 2, 7, 6, 1, 0, 5, 4, 19, 18, 23, 22, 17, 16, 21, 20, 11, 10, 15, 14, 9, 8, 13, 12, 27, 26, 31, 30, 25, 24, 29, 28),
(3, 30): (3, 2, 1, 0, 11, 10, 9, 8, 19, 18, 17, 16, 27, 26, 25, 24, 7, 6, 5, 4, 15, 14, 13, 12, 23, 22, 21, 20, 31, 30, 29, 28),
(3, 32): (3, 2, 7, 6, 11, 10, 15, 14, 19, 18, 23, 22, 27, 26, 31, 30, 1, 0, 5, 4, 9, 8, 13, 12, 17, 16, 21, 20, 25, 24, 29, 28),
(3, 40): (3, 7, 11, 15, 2, 6, 10, 14, 19, 23, 27, 31, 18, 22, 26, 30, 1, 5, 9, 13, 0, 4, 8, 12, 17, 21, 25, 29, 16, 20, 24, 28),
(7, 0): (7, 6, 5, 4, 3, 2, 1, 0, 15, 14, 13, 12, 11, 10, 9, 8, 23, 22, 21, 20, 19, 18, 17, 16, 31, 30, 29, 28, 27, 26, 25, 24),
(7, 6): (7, 6, 5, 4, 15, 14, 13, 12, 3, 2, 1, 0, 11, 10, 9, 8, 23, 22, 21, 20, 31, 30, 29, 28, 19, 18, 17, 16, 27, 26, 25, 24),
(7, 24): (7, 6, 5, 4, 3, 2, 1, 0, 23, 22, 21, 20, 19, 18, 17, 16, 15, 14, 13, 12, 11, 10, 9, 8, 31, 30, 29, 28, 27, 26, 25, 24),
(7, 30): (7, 6, 5, 4, 15, 14, 13, 12, 23, 22, 21, 20, 31, 30, 29, 28, 3, 2, 1, 0, 11, 10, 9, 8, 19, 18, 17, 16, 27, 26, 25, 24),
(7, 60): (7, 6, 15, 14, 23, 22, 31, 30, 5, 4, 13, 12, 21, 20, 29, 28, 3, 2, 11, 10, 19, 18, 27, 26, 1, 0, 9, 8, 17, 16, 25, 24),
(15, 0): (15, 14, 13, 12, 11, 10, 9, 8, 7, 6, 5, 4, 3, 2, 1, 0, 31, 30, 29, 28, 27, 26, 25, 24, 23, 22, 21, 20, 19, 18, 17, 16),
(15, 24): (15, 14, 13, 12, 11, 10, 9, 8, 31, 30, 29, 28, 27, 26, 25, 24, 7, 6, 5, 4, 3, 2, 1, 0, 23, 22, 21, 20, 19, 18, 17, 16),
(31, 0): (31, 30, 29, 28, 27, 26, 25, 24, 23, 22, 21, 20, 19, 18, 17, 16, 15, 14, 13, 12, 11, 10, 9, 8, 7, 6, 5, 4, 3, 2, 1, 0)
}
|
Subgroups
editOh has 98 individual subgroups, which are all shown in the list below. (A Python dictionary of them can be found here.)
They naturally divide in 33 bundles of similar subgroups, whose elements belong to the same conjugacy classes.
In this article these bundles are given naive names based on some of the colors assigned to their elements (like Dih4 green orange).
Each of them has a collapsible box below, containing representations of the individual subgroups.
These belong to 25 bigger bundles, which can be identified with a label in Schoenflies or Coxeter notation (like D2d or [2+,4]).
Each of them has a vertex in the big Hasse diagram below.
Four different kinds of Coxeter notation can be distinguished, based on where they contain plus signs:
| [...]+ | rotate |
| [...] | reflect |
| [...+,...+] | cross |
| [...+, ...] | mixed |
Hasse diagrams
edit| All 25 bundles of similar subgroups |
|---|
 |
| chiral tetrahedral and pyritohedral subgroups | |
|---|---|
 |
 |
List of all subgroups
edit- For the same list including all permutations of the respective example solids, see Full octahedral group/List of all subgroups.
The group itself
edit| Oh S4 × C2 [4,3] | ||
|---|---|---|

|

|
 |
 
| ||
Subgroups of order 24
edit| Td S4 green orange [3,3] | ||
|---|---|---|

|

|
 |
 
| ||
| O S4 blue red [4,3]+ | ||
|---|---|---|

|

|
 |
 
| ||
| Th A4 × C2 [3+,4] | ||
|---|---|---|

|

|
 |
 
| ||
Subgroups of order 16
edit| D4h Dih4 × C2 [2,4] | |||
|---|---|---|---|

|

|

|

|
 
|
 
|
 
| |
Subgroups of order 12
edit| T A4 [3,3]+ | ||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|

|

|
 | ||||||||||||||||||||||
 
| ||||||||||||||||||||||||
| ||||||||||||||||||||||||
| D3d Dih6 [2+,6] | |||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|

|

|

|

|

| |||||||||||||||||||||||
 
|
 
|
 
|
 
| ||||||||||||||||||||||||
| |||||||||||||||||||||||||||
Subgroups of order 8
edit| D2h C23 white [2,2] | |
|---|---|

|

|
 
| |
| D2h C23 green [2,2] | |||
|---|---|---|---|

|

|

|

|
 
|
 
|
 
| |
(Below the C23 subgroups are shown in more detail.)
| C4h C4 × C2 [4+,2] | |||
|---|---|---|---|

|

|

|

|
 
|
 
|
 
| |
| C4v Dih4 green red [4] | |||||||||||||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|

|

|

|

| ||||||||||||||||||||||||||||||||||
 
|
 
|
 
| |||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||
| D2d Dih4 blue orange [2+,4] | |||
|---|---|---|---|

|

|

|

|
 
|
 
|
 
| |
| D2d Dih4 green orange [2+,4] | |||
|---|---|---|---|

|

|

|

|
 | | 
|
 | | 
|
 | | 
| |
| D4 Dih4 blue red [2,4]+ | |||
|---|---|---|---|

|

|

|

|
 
|
 
|
 
| |
(Below the Dih4 subgroups are shown in more detail.)
Subgroups of order 6
edit| C3v S3 green [3] | ||||
|---|---|---|---|---|

|

|

|

|

|
 
|
 
|
 
|
 
| |
| D3 S3 blue [2,3]+ | ||||
|---|---|---|---|---|

|

|

|

|

|
 
|
 
|
 
|
 
| |
(Below the S3 subgroups are shown in more detail.)
| S6 C6 [2+,6+] | ||||
|---|---|---|---|---|

|

|

|

|

|
 
|
 
|
 
|
 
| |
Subgroups of order 4
edit| S4 C4 orange [2+,4+] | |||
|---|---|---|---|

|

|

|

|
 
|
 
|
 
| |
| C4 C4 red [4]+ | |||
|---|---|---|---|

|

|

|

|
 
|
 
|
 
| |
| C2h = D1d V inv white [2+,2] | |||
|---|---|---|---|

|

|

|

|
 
|
 
|
 
| |
| C2h = D1d V inv green [2+,2] | ||||||
|---|---|---|---|---|---|---|

|

|

|

|

|

|

|

|

|

|

|

|

| |

|

|

|

|

|

| |
| C2v V green blue yellow [2] | ||||||
|---|---|---|---|---|---|---|

|

|

|

|

|

|

|

|

|

|

|

|

| |

|

|

|

|

|

| |
| C2v V yellow white [2] | |||
|---|---|---|---|

|

|

|

|
 
|
 
|
 
| |
| C2v V green white [2] | |||
|---|---|---|---|

|

|

|

|
 
|
 
|
 
| |
| D2 V blue white [2,2]+ | |||
|---|---|---|---|

|

|

|

|
 
|
 
|
 
| |
| D2 V white [2,2]+ | |
|---|---|

|

|
 
| |
Subgroups of order 3
edit| C3 C3 [3]+ | ||||
|---|---|---|---|---|

|

|

|

|

|
 
|
 
|
 
|
 
| |
Subgroups of order 2
edit| S2 C2 inv [2+,2+] | |
|---|---|

|

|
 
| |
| Cs = C1v C2 yellow [ ] | |||
|---|---|---|---|

|

|

|

|
 
|
 
|
 
| |
| Cs = C1v C2 green [ ] | ||||||
|---|---|---|---|---|---|---|

|

|

|

|

|

|

|

|

|

|

|

|

| |

|

|

|

|

|

| |
| C2 C2 white [2]+ | |||
|---|---|---|---|

|

|

|

|
 
|
 
|
 
| |
| C2 C2 blue [2]+ | ||||||
|---|---|---|---|---|---|---|

|

|

|

|

|

|

|

|

|

|

|

|

| |

|

|

|

|

|

| |
The trivial group
edit| C1 C1 [ ]+ | |
|---|---|

|
|
 
| |
Different appearances of the same group
editSymmetry group of the cuboid
editThe symmetry group of the cuboid C23 appears in two essentially different ways as a subgroup of Oh.
The one where the cuboid is the cube itself is the most intuitive one.
In the other one the cuboid is the original cube rotated by 45° around an axis. The one where it is rotated around the z-axis is shown below.
There are 4 individual subgroups (see above).
| D2h C23 white [2,2] | ||||||||
|---|---|---|---|---|---|---|---|---|

|
 |
 |
 |
 |
 |
 |
 |
 |
| D2h C23 green [2,2] | ||||||||
|---|---|---|---|---|---|---|---|---|

|
 |
 |
 |
 |
 |
 |
 |
 |
Symmetry group of the square
editThe symmetry group of the square appears in four essentially different ways as a subgroup of Oh. (C4v or [4] being the most intuitive among them.)
There are 12 individual Dih4 subgroups (see above). Shown below are the four where the square is seen "from above", i.e. a point on the positive z-axis.
In the white box above the colored boxes of the four subgroups are the permutations of the square. Their 2×2 transformation matrices are the top left submatrices of the four 3×3 matrices in the same column. So the last non-zero entry of the 3×3 matrix determines the permutation in this column. (So each column has only two different permutations.) The pattern of these eight last entries identifies the subgroup. It is shown on the left in the little 4×2 matrix under the example solid.
| Square permutations for comparison | ||||||||
|---|---|---|---|---|---|---|---|---|

|
 |
 |
 |
 |
 |
 |
 |
 |
| C4v Dih4 green red [4] | ||||||||
|---|---|---|---|---|---|---|---|---|
 |
 |
 |
 |
 |
 |
 |
 |
 |
| D2d Dih4 blue orange [2+,4] | ||||||||
|---|---|---|---|---|---|---|---|---|
 |
 |
 |
 |
 |
 |
 |
 |
 |
| D2d Dih4 green orange [2+,4] | ||||||||
|---|---|---|---|---|---|---|---|---|
 |
 |
 |
 |
 |
 |
 |
 |
 |
| D4 Dih4 blue red [2,4]+ | ||||||||
|---|---|---|---|---|---|---|---|---|
 |
 |
 |
 |
 |
 |
 |
 |
 |
Symmetry group of the triangle
editThe symmetry group of the triangle appears in two essentially different ways as a subgroup of Oh, with C3v or [3] being the most intuitive among them.
There are 8 individual subgroups (see above). Shown below are the ones where the triangle is seen from a point on the negative main diagonal of the coordinate system.
| C3v S3 green [3] | ||||||
|---|---|---|---|---|---|---|

|
 |
 |
 |
 |
 |
 |
| D3 S3 blue [2,3]+ | ||||||
|---|---|---|---|---|---|---|

|
 |
 |
 |
 |
 |
 |
Cuboctahedral example solids and contained hexagons
edit
|
Code
editThe Python code used to create many of the illustrations in this article can be found here: https://github.com/watchduck/full_octahedral_group
The following code shows bijections from pairs to other representations:
| S4 based identifiers |
|---|
|
is represented as . (0, 0): 0, (0, 1): 1, (0, 2): 2, (0, 3): 3, (0, 4): 4, (0, 5): 5, (1, 0): 47, (1, 1): 46, (1, 2): 45, (1, 3): 44, (1, 4): 43, (1, 5): 42, (2, 0): 40, (2, 1): 41, (2, 2): 37, (2, 3): 36, (2, 4): 39, (2, 5): 38, (3, 0): 7, (3, 1): 6, (3, 2): 10, (3, 3): 11, (3, 4): 8, (3, 5): 9, (4, 0): 31, (4, 1): 30, (4, 2): 34, (4, 3): 35, (4, 4): 32, (4, 5): 33, (5, 0): 16, (5, 1): 17, (5, 2): 13, (5, 3): 12, (5, 4): 15, (5, 5): 14, (6, 0): 23, (6, 1): 22, (6, 2): 21, (6, 3): 20, (6, 4): 19, (6, 5): 18, (7, 0): 24, (7, 1): 25, (7, 2): 26, (7, 3): 27, (7, 4): 28, (7, 5): 29 |
| Permutations of cube vertices |
|---|
|
The initial order is (0, 0): (0, 1, 2, 3, 4, 5, 6, 7), (0, 1): (0, 2, 1, 3, 4, 6, 5, 7), (0, 2): (0, 1, 4, 5, 2, 3, 6, 7), (0, 3): (0, 4, 1, 5, 2, 6, 3, 7), (0, 4): (0, 2, 4, 6, 1, 3, 5, 7), (0, 5): (0, 4, 2, 6, 1, 5, 3, 7), (1, 0): (1, 0, 3, 2, 5, 4, 7, 6), (1, 1): (1, 3, 0, 2, 5, 7, 4, 6), (1, 2): (1, 0, 5, 4, 3, 2, 7, 6), (1, 3): (1, 5, 0, 4, 3, 7, 2, 6), (1, 4): (1, 3, 5, 7, 0, 2, 4, 6), (1, 5): (1, 5, 3, 7, 0, 4, 2, 6), (2, 0): (2, 3, 0, 1, 6, 7, 4, 5), (2, 1): (2, 0, 3, 1, 6, 4, 7, 5), (2, 2): (2, 3, 6, 7, 0, 1, 4, 5), (2, 3): (2, 6, 3, 7, 0, 4, 1, 5), (2, 4): (2, 0, 6, 4, 3, 1, 7, 5), (2, 5): (2, 6, 0, 4, 3, 7, 1, 5), (3, 0): (3, 2, 1, 0, 7, 6, 5, 4), (3, 1): (3, 1, 2, 0, 7, 5, 6, 4), (3, 2): (3, 2, 7, 6, 1, 0, 5, 4), (3, 3): (3, 7, 2, 6, 1, 5, 0, 4), (3, 4): (3, 1, 7, 5, 2, 0, 6, 4), (3, 5): (3, 7, 1, 5, 2, 6, 0, 4), (4, 0): (4, 5, 6, 7, 0, 1, 2, 3), (4, 1): (4, 6, 5, 7, 0, 2, 1, 3), (4, 2): (4, 5, 0, 1, 6, 7, 2, 3), (4, 3): (4, 0, 5, 1, 6, 2, 7, 3), (4, 4): (4, 6, 0, 2, 5, 7, 1, 3), (4, 5): (4, 0, 6, 2, 5, 1, 7, 3), (5, 0): (5, 4, 7, 6, 1, 0, 3, 2), (5, 1): (5, 7, 4, 6, 1, 3, 0, 2), (5, 2): (5, 4, 1, 0, 7, 6, 3, 2), (5, 3): (5, 1, 4, 0, 7, 3, 6, 2), (5, 4): (5, 7, 1, 3, 4, 6, 0, 2), (5, 5): (5, 1, 7, 3, 4, 0, 6, 2), (6, 0): (6, 7, 4, 5, 2, 3, 0, 1), (6, 1): (6, 4, 7, 5, 2, 0, 3, 1), (6, 2): (6, 7, 2, 3, 4, 5, 0, 1), (6, 3): (6, 2, 7, 3, 4, 0, 5, 1), (6, 4): (6, 4, 2, 0, 7, 5, 3, 1), (6, 5): (6, 2, 4, 0, 7, 3, 5, 1), (7, 0): (7, 6, 5, 4, 3, 2, 1, 0), (7, 1): (7, 5, 6, 4, 3, 1, 2, 0), (7, 2): (7, 6, 3, 2, 5, 4, 1, 0), (7, 3): (7, 3, 6, 2, 5, 1, 4, 0), (7, 4): (7, 5, 3, 1, 6, 4, 2, 0), (7, 5): (7, 3, 5, 1, 6, 2, 4, 0) |
| Permutations of octahedron vertices |
|---|
|
The initial order is (0, 0): (0, 1, 2, 3, 4, 5), (0, 1): (2, 3, 0, 1, 4, 5), (0, 2): (0, 1, 4, 5, 2, 3), (0, 3): (4, 5, 0, 1, 2, 3), (0, 4): (2, 3, 4, 5, 0, 1), (0, 5): (4, 5, 2, 3, 0, 1), (1, 0): (1, 0, 2, 3, 4, 5), (1, 1): (2, 3, 1, 0, 4, 5), (1, 2): (1, 0, 4, 5, 2, 3), (1, 3): (4, 5, 1, 0, 2, 3), (1, 4): (2, 3, 4, 5, 1, 0), (1, 5): (4, 5, 2, 3, 1, 0), (2, 0): (0, 1, 3, 2, 4, 5), (2, 1): (3, 2, 0, 1, 4, 5), (2, 2): (0, 1, 4, 5, 3, 2), (2, 3): (4, 5, 0, 1, 3, 2), (2, 4): (3, 2, 4, 5, 0, 1), (2, 5): (4, 5, 3, 2, 0, 1), (3, 0): (1, 0, 3, 2, 4, 5), (3, 1): (3, 2, 1, 0, 4, 5), (3, 2): (1, 0, 4, 5, 3, 2), (3, 3): (4, 5, 1, 0, 3, 2), (3, 4): (3, 2, 4, 5, 1, 0), (3, 5): (4, 5, 3, 2, 1, 0), (4, 0): (0, 1, 2, 3, 5, 4), (4, 1): (2, 3, 0, 1, 5, 4), (4, 2): (0, 1, 5, 4, 2, 3), (4, 3): (5, 4, 0, 1, 2, 3), (4, 4): (2, 3, 5, 4, 0, 1), (4, 5): (5, 4, 2, 3, 0, 1), (5, 0): (1, 0, 2, 3, 5, 4), (5, 1): (2, 3, 1, 0, 5, 4), (5, 2): (1, 0, 5, 4, 2, 3), (5, 3): (5, 4, 1, 0, 2, 3), (5, 4): (2, 3, 5, 4, 1, 0), (5, 5): (5, 4, 2, 3, 1, 0), (6, 0): (0, 1, 3, 2, 5, 4), (6, 1): (3, 2, 0, 1, 5, 4), (6, 2): (0, 1, 5, 4, 3, 2), (6, 3): (5, 4, 0, 1, 3, 2), (6, 4): (3, 2, 5, 4, 0, 1), (6, 5): (5, 4, 3, 2, 0, 1), (7, 0): (1, 0, 3, 2, 5, 4), (7, 1): (3, 2, 1, 0, 5, 4), (7, 2): (1, 0, 5, 4, 3, 2), (7, 3): (5, 4, 1, 0, 3, 2), (7, 4): (3, 2, 5, 4, 1, 0), (7, 5): (5, 4, 3, 2, 1, 0) |
| Pairs in reverse colex order | |
|---|---|
|
The reverse colexicographic order of permutations is the order of their respective integer values. | |
| cube | octahedron |
(0, 0), (0, 2), (0, 1), (0, 4), (0, 3), (0, 5), (1, 0), (1, 2), (1, 1), (1, 4), (1, 3), (1, 5), (2, 1), (2, 4), (2, 0), (2, 2), (2, 5), (2, 3), (3, 1), (3, 4), (3, 0), (3, 2), (3, 5), (3, 3), (4, 3), (4, 5), (4, 2), (4, 0), (4, 4), (4, 1), (5, 3), (5, 5), (5, 2), (5, 0), (5, 4), (5, 1), (6, 5), (6, 3), (6, 4), (6, 1), (6, 2), (6, 0), (7, 5), (7, 3), (7, 4), (7, 1), (7, 2), (7, 0) |
(0, 0), (1, 0), (2, 0), (3, 0), (0, 1), (2, 1), (1, 1), (3, 1), (4, 0), (5, 0), (6, 0), (7, 0), (4, 1), (6, 1), (5, 1), (7, 1), (0, 2), (1, 2), (4, 2), (5, 2), (0, 3), (4, 3), (1, 3), (5, 3), (2, 2), (3, 2), (6, 2), (7, 2), (2, 3), (6, 3), (3, 3), (7, 3), (0, 4), (2, 4), (4, 4), (6, 4), (0, 5), (4, 5), (2, 5), (6, 5), (1, 4), (3, 4), (5, 4), (7, 4), (1, 5), (5, 5), (3, 5), (7, 5) |
| 3×3 matrices |
|---|
(0, 0): [[ 1, 0, 0], [0, 1, 0], [0, 0, 1]], (0, 1): [[0, 1, 0], [ 1, 0, 0], [0, 0, 1]], (0, 2): [[ 1, 0, 0], [0, 0, 1], [0, 1, 0]], (0, 3): [[0, 1, 0], [0, 0, 1], [ 1, 0, 0]], (0, 4): [[0, 0, 1], [ 1, 0, 0], [0, 1, 0]], (0, 5): [[0, 0, 1], [0, 1, 0], [ 1, 0, 0]], (1, 0): [[-1, 0, 0], [0, 1, 0], [0, 0, 1]], (1, 1): [[0,-1, 0], [ 1, 0, 0], [0, 0, 1]], (1, 2): [[-1, 0, 0], [0, 0, 1], [0, 1, 0]], (1, 3): [[0,-1, 0], [0, 0, 1], [ 1, 0, 0]], (1, 4): [[0, 0,-1], [ 1, 0, 0], [0, 1, 0]], (1, 5): [[0, 0,-1], [0, 1, 0], [ 1, 0, 0]], (2, 0): [[ 1, 0, 0], [0,-1, 0], [0, 0, 1]], (2, 1): [[0, 1, 0], [-1, 0, 0], [0, 0, 1]], (2, 2): [[ 1, 0, 0], [0, 0,-1], [0, 1, 0]], (2, 3): [[0, 1, 0], [0, 0,-1], [ 1, 0, 0]], (2, 4): [[0, 0, 1], [-1, 0, 0], [0, 1, 0]], (2, 5): [[0, 0, 1], [0,-1, 0], [ 1, 0, 0]], (3, 0): [[-1, 0, 0], [0,-1, 0], [0, 0, 1]], (3, 1): [[0,-1, 0], [-1, 0, 0], [0, 0, 1]], (3, 2): [[-1, 0, 0], [0, 0,-1], [0, 1, 0]], (3, 3): [[0,-1, 0], [0, 0,-1], [ 1, 0, 0]], (3, 4): [[0, 0,-1], [-1, 0, 0], [0, 1, 0]], (3, 5): [[0, 0,-1], [0,-1, 0], [ 1, 0, 0]], (4, 0): [[ 1, 0, 0], [0, 1, 0], [0, 0,-1]], (4, 1): [[0, 1, 0], [ 1, 0, 0], [0, 0,-1]], (4, 2): [[ 1, 0, 0], [0, 0, 1], [0,-1, 0]], (4, 3): [[0, 1, 0], [0, 0, 1], [-1, 0, 0]], (4, 4): [[0, 0, 1], [ 1, 0, 0], [0,-1, 0]], (4, 5): [[0, 0, 1], [0, 1, 0], [-1, 0, 0]], (5, 0): [[-1, 0, 0], [0, 1, 0], [0, 0,-1]], (5, 1): [[0,-1, 0], [ 1, 0, 0], [0, 0,-1]], (5, 2): [[-1, 0, 0], [0, 0, 1], [0,-1, 0]], (5, 3): [[0,-1, 0], [0, 0, 1], [-1, 0, 0]], (5, 4): [[0, 0,-1], [ 1, 0, 0], [0,-1, 0]], (5, 5): [[0, 0,-1], [0, 1, 0], [-1, 0, 0]], (6, 0): [[ 1, 0, 0], [0,-1, 0], [0, 0,-1]], (6, 1): [[0, 1, 0], [-1, 0, 0], [0, 0,-1]], (6, 2): [[ 1, 0, 0], [0, 0,-1], [0,-1, 0]], (6, 3): [[0, 1, 0], [0, 0,-1], [-1, 0, 0]], (6, 4): [[0, 0, 1], [-1, 0, 0], [0,-1, 0]], (6, 5): [[0, 0, 1], [0,-1, 0], [-1, 0, 0]], (7, 0): [[-1, 0, 0], [0,-1, 0], [0, 0,-1]], (7, 1): [[0,-1, 0], [-1, 0, 0], [0, 0,-1]], (7, 2): [[-1, 0, 0], [0, 0,-1], [0,-1, 0]], (7, 3): [[0,-1, 0], [0, 0,-1], [-1, 0, 0]], (7, 4): [[0, 0,-1], [-1, 0, 0], [0,-1, 0]], (7, 5): [[0, 0,-1], [0,-1, 0], [-1, 0, 0]] |
| Truncated cuboctahedron coordinates |
|---|
|
The points of the truncated cuboctahedron are the permutations of . a = sqrt(2) + 1 b = 2*sqrt(2) + 1 (0, 0): (-b, -a, -1), (0, 1): (-a, -b, -1), (0, 2): (-b, -1, -a), (0, 3): (-a, -1, -b), (0, 4): (-1, -b, -a), (0, 5): (-1, -a, -b), (1, 0): ( b, -a, -1), (1, 1): ( a, -b, -1), (1, 2): ( b, -1, -a), (1, 3): ( a, -1, -b), (1, 4): ( 1, -b, -a), (1, 5): ( 1, -a, -b), (2, 0): (-b, a, -1), (2, 1): (-a, b, -1), (2, 2): (-b, 1, -a), (2, 3): (-a, 1, -b), (2, 4): (-1, b, -a), (2, 5): (-1, a, -b), (3, 0): ( b, a, -1), (3, 1): ( a, b, -1), (3, 2): ( b, 1, -a), (3, 3): ( a, 1, -b), (3, 4): ( 1, b, -a), (3, 5): ( 1, a, -b), (4, 0): (-b, -a, 1), (4, 1): (-a, -b, 1), (4, 2): (-b, -1, a), (4, 3): (-a, -1, b), (4, 4): (-1, -b, a), (4, 5): (-1, -a, b), (5, 0): ( b, -a, 1), (5, 1): ( a, -b, 1), (5, 2): ( b, -1, a), (5, 3): ( a, -1, b), (5, 4): ( 1, -b, a), (5, 5): ( 1, -a, b), (6, 0): (-b, a, 1), (6, 1): (-a, b, 1), (6, 2): (-b, 1, a), (6, 3): (-a, 1, b), (6, 4): (-1, b, a), (6, 5): (-1, a, b), (7, 0): ( b, a, 1), (7, 1): ( a, b, 1), (7, 2): ( b, 1, a), (7, 3): ( a, 1, b), (7, 4): ( 1, b, a), (7, 5): ( 1, a, b) |
These are Python dictionaries without the surrounding braces. (Dicts work only in one direction, but bidict can be used to get back to the pairs.)
A dictionary of these permutations and their properties (including conjugacy class and inverse) can be found here.
A dictionary of all the subgroups can be found here (as a bijection from naive names to tuples of S4 based numbers).
































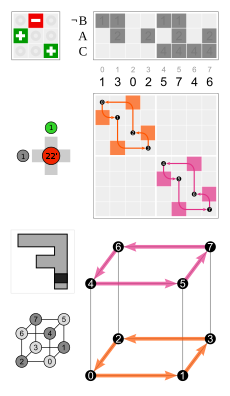













![{\displaystyle pm=\mathrm {cvp} (am,an)[bm]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/62eaa1dc0b24c376d0d96e42cd44c7a90caa8781)



![{\displaystyle pm=\mathrm {cvp} (2,3)[6]=1}](https://wikimedia.org/api/rest_v1/media/math/render/svg/dd450a64b3aa87a36bc3fed03df997a303205fdb)


































































































































































































































































































































































































