Astronomy college course/Sizes of white dwarfs, neutron stars, quasars
This unit explores how know the sizes of white dwarfs, neutron stars, and quasars. In the process of answering these questions, we shall learn about the doppler effect in astronomy, the gravitational redshift, energy conservation, the cosmic redshift, and causality.
-
Redshift and blueshift can be associated with the Doppler effect
-
redshifted spectral lines
-
High-redshift galaxies (Hubble telescope) [1]
-
Most white dwarfs have radius of .01R☉, but the more massive ones can be as small as 0.002R☉.
White dwarfs and the gravitational redshift
editReview white dwarf stars:
- The fate of stars with less than 1.4 solar masses (includes sun)
- Radius of 0.01R☉ (i.e. 0.01 Solar radii) can be deduced from its position on the HR diagram.
- We know the luminosity because we know the apparent magnitudes and the distance, which allows us to apply the inverse square law to deduce total power output (also called luminosity). But how do we know the distance? That was easy, the first discovered white dwarf was observed as a companion to a well known star.
- And because we know the distance to the companion star, we also know that mass of the white dwarf (if we believe in gravity)
- , where P is the period of orbit in years, and a is the semi-major axis measured in AU. The net mass, , is the sum of the mass of both bodies, and is normalized to the mass of the Sun. For a planet of mass, , orbiting a star of much larger mass, , the normalized net mass is . The mass of the Sun, , is 1.99×1030 kilograms. If for some object, then that object is twice as massive as the Sun. One year is 3.15×107 seconds.
- But are we really sure about the temperature? The equations for luminosity, color, temperature, distance and relative magnitude all assume that the star is a black body. How do we know that it is a black body?


- is Wein's law that relates the peak emission wavelength, λmax, of a black body to temperature, T measured in Kelvins. Peak wavelength, λmax, is measured in nanometers (1nm=10-9m). If temperature is measured in units normalized to the Sun's temperature, , then
- where is the temperature normalized to the Sun's temperature.
The Stefan-Boltzmann law is usually written as P=σAT4, where A is surface area, T is temperature (in Kelvins), and σ is the Stefan-Boltzmann constant. The power, P, can be written as normalized luminosity, , where L☉ =3.85×1026W is the power output (or luminosity) of the Sun. In these normalized units, the Stefan-Boltzmann law is:
- , where is the radius and temperature normalized to the Sun's radius and is the temperature normalized to the Sun's temperature.
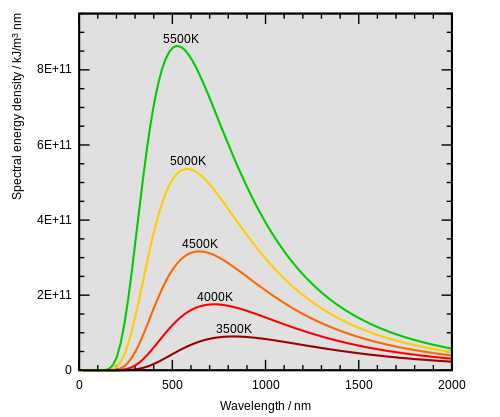
- is a "normalized intensity", closely related to relative magnitude, that allows students to combine equations and solve problems without resorting to the logarithmic magnitude scale. If the distance to the stellar object, D, is measured in parsecs, it is the power per square parsec that enters a telescope on Earth. The luminosity, , (in solar units) is a measure of the absolute magnitude. In general, is the inverse-square law.
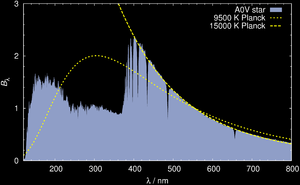
- The figure to the right shows the actual spectrum of the type A0V star Vega. Stars don't really have a unique temperature because we see through several layers that are semi-transparent, allowing light from a variety of temperatures to reach our eyes (telescope). By convention, astronomers have devised an "effective temperature", and for Vega, it is 9500K. Reality is complicated. Even humans have different temperatures for different parts of the body.
Gravitational redshift
edit
- Another verification of the white dwarf's size comes from General Relativity (which is much more advanced than special relativity because it demands that Euclidean geometry be abandoned).
Time dilation is one of the bewildering features of relativity.

An intuitive understanding of the gravitational redshift comes from the photon picture of light and conservation of energy. When a particle of mass m is ejected from a massive planet (of mass M), the particle loses kinetic energy, KE =mv2 , where v is speed. By Einstein's theory of special relativity, the speed of the particle, v, never exceeds the speed of light, c (where c≈ 3×108m/s). But a photon has zero mass and a speed equal to c (if it is in a vacuum). Since the energy of a photon is given by E=hf, the only way a photon can lose energy is to reduce it's frequency, f (since h is a universal constant called Planck's constant).
Time dilation is one of the bewildering features of relativity. The light clock shown illustrates how Einstein deduce that a moving clock seems to travel slowly. The "tick" of this clock is the time it takes for light to bounce from one mirror to the other. If all observers perceive light to travel from one mirror to the other, and if a stationary and moving observer both perceive light to be travelling at the same speed, then the "tick" of the clock seems slower to an observer watching a moving clock. (This would not be a paradox, for example of people tossing a ball back and forth on a train, since the two observers perceive different speeds for the ball.)
- Excellent images and schematic of Crab
- Quantum calculations of the radius of a white dwarf Very advanced from an upper division or graduate level course in astrophysics, but see the graph of theoretical radius versus mass. Some understandable sections include:
- White dwarf radius calculations 520-22 (18-20)
- White dwarf electron pressure versus ion pressure , 531 (29)
- Neutron star internal structure 540 (38)
- Neutron star Lighthouse effect 543-545 (41-43)
- Light pules from Crab center, "glitches" 548-550 (46-48)
- Hypothesis for fast pulsars 553 (51)
- Ideal Fermi gas (Wikipedia article stating similarity of electrons in a crystal to those in a white dwarf)
- Identified in 1731 by John Bevis; independently rediscovered in 1758 by Charles Messier as M1 (who was hunting comets and eventually made a catalog of things that are not comets (things comet hunters should ignore). (See Messier objects)
- Blue color produced by synchrotron radiation, which is radiation given off by the curving motion of electrons in a magnetic field.
- Even though the Crab Nebula is the focus of much attention among astronomers, its distance remains an open question, owing to uncertainties in every method used to estimate its distance. In 2008, the consensus was that its distance from Earth is 2.0 ± 0.5 kpc (6.5 ± 1.6 kly). Note: It is best to remember percent uncertainty: 0.5/2.0 = 0.25/1 = 0.25 =25%.
- One method of calculating the distance to compare the angular expansion with the spectroscopically determined expansion velocity.
Video of a lava lamp that resembles both a Salt dome, as well as a Corona on Venus or Miranda (moon). - The "fingers" seen in close-up images are caused by a low density gas pushing against a high density gas that is essential "above". Such a combinations of fluids is unstable. See also Bill Blair's page.
How we know neutron stars are small
edit- The plasma surrounding the neutron star is far too unstable to model it as a simple black body. The position on the HR diagram tells us nothing. As far as I can tell, we have three reasons for believing the small size:
- The size can be calculated using an advanced version of Quantum Theory. This calculation is less reliable than for the white dwarf because nuclear physics and relativity play a greater role. Is the theoretical calculation just slightly less reliable or much less reliable? Few people have the knowledge to answer that question with any authority.
- The rapid rotation of the pulsar renders it impossible for it to be a white dwarf (remember white dwarfs are simpler to model -- they would certainly fly apart if they rotated that fast, or so the experts say)
- A very simple energy calculation confirms the theoretical radius of a neutron
- Begin with the theoretical mass and radius of a neutron star.
- Use the observed rotation rate.
- Matter that is in motion has "kinetic energy". In the non-relativistic theory, the kinetic energy, KE=½mv2, where m is the mass of each atom and v is the speed. The relativistic calculation is almost as simple. Using calculus, one can take a solid spinning object (such as a bowling ball) and calculate the total kinetic energy, even though not all the atoms are moving at the same speed (the ones near the center have much less kinetic energy). The result of this calculation is the total kinetic energy of a pulsar. FYI, the formula is, , where M is mass, R is radius, and P is period.
- Another observable fact about pulsars is the rate at which they are slowing down. In other words we know how much energy is being lost by the pulsar every second. This energy has to go somewhere, and the spinning magnet delivers this power (mostly to to the electrons) in the surrounding Crab nebula.
- Finally, the total radiated energy of the Crab nebula has been measured. The Crab is emitting light at all wavelengths. And, it roughly matches the theoretical prediction.
Quasars (supermassive black holes?)
editA very big universe
edit- There are 7 billion people on earth
- If you are "one in a million", there are 7,000 people on Earth just like you.
- If you divided up all the stars in the Milky way among all people alive today, each person on Earth would get about 40 stars.
- There at least half as many galaxies in the universe as there are stars in the Milky Way.
A surprisingly young universe
edit- One in 2.75×1045 are the odds that a monkey typing randomly on a typewriter (lock in capital letters) would type the sentance: "TO BE OR NOT TO BE, THAT IS THE QUESTION."
- Suppose there were 7 billion monkeys for every star in the universe. That would be 4.2×1032 monkeys. If these monkeys typed at a rate of one sentence every second for the age of the universe (13 billion years), then this sentence will get typed approximately 60 times.
- How did life evolve in this short time?
Hubble's Law
editRecall that the standard candle is an object of known luminisoty, which permits distance to be calculated from the relative magnitude (i.e. light intensity as seen from Earth).


![High-redshift galaxies (Hubble telescope) [1]](http://upload.wikimedia.org/wikipedia/commons/thumb/0/0a/High-redshift_galaxy_candidates_in_the_Hubble_Ultra_Deep_Field_2012.jpg/108px-High-redshift_galaxy_candidates_in_the_Hubble_Ultra_Deep_Field_2012.jpg)



















