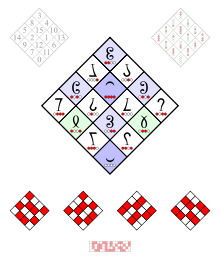
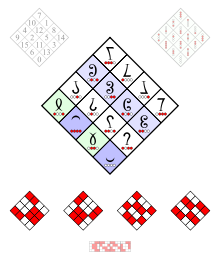
Order 4 magic squares; Walsh permutations
There are 24*9=216 Walsh permutations that correspond to magic squares of order 4.
They have these 9 compression vectors and all their permutations:
| ||
|
5 7 10 11 5 7 10 14 5 7 11 13 5 7 13 14 5 10 11 13 5 10 13 14 7 10 11 14 7 11 13 14 10 11 13 14 |
The following table shows the compression vectors in the RevCoLex order of the permutations.
Half of those of type IV, V, VI are omitted, because they are reflections. Half of those of type I, II, III are reflections, but they are shown.
I  (p=6, x=9)
|
II  (p=9, x=6)
|
III  (p=15, x=15)
|
IV  (p=1, x=4)
|
V  (p=2, x=8)
|
VI  (p=3, x=12)
|
|---|---|---|---|---|---|
11 13 5 10 11 5 13 10 13 11 5 10 5 11 13 10 13 5 11 10 5 13 11 10 11 13 10 5 11 5 10 13 13 11 10 5 5 11 10 13 13 5 10 11 5 13 10 11 11 10 13 5 11 10 5 13 13 10 11 5 5 10 11 13 13 10 5 11 5 10 13 11 10 11 13 5 10 11 5 13 10 13 11 5 10 5 11 13 10 13 5 11 10 5 13 11 |
7 5 14 10 7 5 10 14 5 7 14 10 5 7 10 14 7 14 5 10 7 10 5 14 5 14 7 10 5 10 7 14 14 7 5 10 10 7 5 14 14 5 7 10 10 5 7 14 7 14 10 5 7 10 14 5 5 14 10 7 5 10 14 7 14 7 10 5 10 7 14 5 14 5 10 7 10 5 14 7 14 10 7 5 10 14 7 5 14 10 5 7 10 14 5 7 |
7 11 13 14 11 7 13 14 7 13 11 14 11 13 7 14 13 7 11 14 13 11 7 14 7 11 14 13 11 7 14 13 7 13 14 11 11 13 14 7 13 7 14 11 13 11 14 7 7 14 11 13 11 14 7 13 7 14 13 11 11 14 13 7 13 14 7 11 13 14 11 7 14 7 11 13 14 11 7 13 14 7 13 11 14 11 13 7 14 13 7 11 14 13 11 7 |
7 11 13 5 7 11 5 13 11 7 13 5 11 7 5 13 7 13 11 5 7 5 11 13 11 13 7 5 11 5 7 13 13 7 11 5 5 7 11 13 13 11 7 5 5 11 7 13 7 13 5 11 7 5 13 11 11 13 5 7 11 5 13 7 13 7 5 11 5 7 13 11 13 11 5 7 5 11 13 7 13 5 7 11 5 13 7 11 13 5 11 7 5 13 11 7 |
7 11 14 10 11 7 14 10 7 11 10 14 11 7 10 14 7 14 11 10 11 14 7 10 7 10 11 14 11 10 7 14 14 7 11 10 14 11 7 10 10 7 11 14 10 11 7 14 7 14 10 11 7 10 14 11 11 14 10 7 11 10 14 7 14 7 10 11 10 7 14 11 14 11 10 7 10 11 14 7 14 10 7 11 10 14 7 11 14 10 11 7 10 14 11 7 |
13 5 14 10 5 13 14 10 13 5 10 14 5 13 10 14 13 14 5 10 5 14 13 10 13 10 5 14 5 10 13 14 14 13 5 10 14 5 13 10 10 13 5 14 10 5 13 14 13 14 10 5 5 14 10 13 13 10 14 5 5 10 14 13 14 13 10 5 14 5 10 13 10 13 14 5 10 5 14 13 14 10 13 5 14 10 5 13 10 14 13 5 10 14 5 13 |
 wp(11,13, 5,10) |
 wp(14, 7, 5,10) |
 wp(13,11,14, 7) |
 wp(13,11, 7, 5) |
 wp(14, 7,11,10) |
 wp(10, 5,13,14) |
|
|
|
|
|
|
|
There are 24*81=1944 Walsh permutations that correspond to semi-magic squares of order 4 (where the diagonals do not necessarily sum up to 30).
They have these 81 compression vectors and all their permutations:
5 6 7 9 5 6 7 10 5 6 7 11 5 6 7 13 5 6 7 14 5 6 7 15 5 6 9 11 5 6 9 13 5 6 9 14 5 6 10 11 5 6 10 13 5 6 10 14 5 6 11 15 5 6 13 15 5 6 14 15 5 7 9 10 5 7 9 13 5 7 9 15 5 7 10 11 5 7 10 14 5 7 11 13 5 7 11 15 5 7 13 14 5 7 14 15 5 9 10 11 5 9 10 13 5 9 10 14 5 9 11 13 5 9 11 15 5 9 13 14 5 9 13 15 5 9 14 15 5 10 11 13 5 10 13 14 5 11 13 15 5 13 14 15 6 7 9 10 6 7 9 11 6 7 9 13 6 7 10 14 6 7 10 15 6 7 11 14 6 7 11 15 6 7 13 14 6 7 13 15 6 9 10 11 6 9 10 13 6 9 10 14 6 9 11 14 6 9 13 14 6 10 11 14 6 10 11 15 6 10 13 14 6 10 13 15 6 10 14 15 6 11 14 15 6 13 14 15 7 9 10 11 7 9 10 15 7 9 11 13 7 9 11 15 7 9 13 15 7 10 11 14 7 10 11 15 7 10 14 15 7 11 13 14 7 11 13 15 7 11 14 15 7 13 14 15 9 10 11 13 9 10 11 14 9 10 11 15 9 10 13 15 9 10 14 15 9 11 13 14 9 11 14 15 9 13 14 15 10 11 13 14 10 11 13 15 10 13 14 15 11 13 14 15 |